Benutzer:Duesentrieb/Trigonometrie

Die Formelsammlung zur Trigonometrie ist ein Teil der Formelsammlung, in der auch Formeln der anderen Fachbereiche zu finden sind. Einen Überblick aus anderen Bereichen der Geometrie gibt die Formelsammlung Geometrie.

Aus der Formelsammlung Geometrie#Dreieck

Das Dreieck

• Benennung der Seiten und Winkel

Der Innenwinkel beim Eckpunkt A nennt man $\alpha$ (griechische Kleinbuchstaben) Die Dreiecksseite (bzw. deren Länge) gegenüber der Ecke A nennt man a

• Gleichseitiges Dreieck

Alle Seiten sind gleich lang Alle Winkel sind gleich groß (60°) Höhenlinien = Symmetrieachsen = Winkelhalbierende = Seitenhalbierende

• Gleichschenkliges Dreieck

2 Seiten sind gleichlang (Schenkel a und b) Die zwei Basiswinkel ($\alpha$ und $\beta$)sind gleich groß Die Höhenlinie halbiert den Winkel $\gamma$ Die Höhenlinie halbiert die Basis c

• Rechwinkliges Dreieck

$\alpha$ + $\beta$ = 90° Hypotenuse = längste Seite = Seite gegenüber 90°-Winkel Satz des Pythagoras: (Kathete a)2 + (Kathete b)2 = (Hypotenuse c)2

• Schnittpunkt der Seitenhalbierenden

Die Seitenhalbierenden sind die Schwerelinien Die Seitenhalbierenden schneiden sich in einem Punkt, dem Schwerpunkt S.

• Schnittpunkt der Mittelsenkrechten

1. Der Schnittpunkt der Mittelsenkrechten entspricht dem Mittelpunkt des Umkreises.

• Schnittpunkt der Winkelhalbierenden

Der Schnittpunkt der Winkelhalbierenden entspricht dem Mittelpunkt des Inkreises. w$\alpha$ ist die Winkelhalbierende des Winkels $\alpha$.

• Höhenschnittpunkt

Die Höhen schneiden sich in einem Punkt. Die Höhe hc ist die Höhe vom Punkt C aus auf die Seite c. D ist der Höhenfußpunkt von hc.

• Flächenberechnung mit Grundseite und Höhe

$A={\frac {g\cdot h}{2}}$

• Flächenberechnung mit einem Winkel

$A={\frac {b\cdot c\cdot \sin(\alpha )}{2}}$

(b und c sind die den Winkel $\alpha$ einschließenden Seiten)

Satzgruppe des Pythagoras

• Satz des Pythagoras

Im rechtwinkligen Dreieck ist die Fläche des Quadrats über der Hypothenuse gleich der Summe der Flächen der Quadrate über den Katheten:

$\mathbf {a^{2}+b^{2}=c^{2}}$

• Kathetensatz

Im rechtwinkligen Dreieck ist das Quadrat über einer Kathete flächengleich dem Rechteck aus der Hypothenuse und der Projektion dieser Kathete auf die Hypothenuse:

$a^{2}=p\cdot c,\ b^{2}=q\cdot c$

• Höhensatz

Im rechtwinkligen Dreieck ist das Quadrat über der Höhe auf der Hypothenuse flächengleich mit dem Rechteck aus den Hypothenusenabschnitten.

$h^{2}=q\cdot p$

---

---

Von Formelsammlung Geometrie#Trigonometrie

Trigonometrische Funktionen

Definitionen

• Sinus

$\sin \alpha ={Gegenkathete \over Hypotenuse}={a \over c}$

• Cosinus

$\cos \alpha ={Ankathete \over Hypotenuse}={b \over c}$

• Tangens

$\tan \alpha ={Gegenkathete \over Ankathete}={a \over b}$

Eigenschaften von Sinus, Kosinus und Tangens

$\sin ^{2}(\alpha )+\cos ^{2}(\alpha )=1$

$\tan(\alpha )={\frac {\sin(\alpha )}{\cos(\alpha )}}$

$\sin(\alpha )=\cos(90^{\circ }-\alpha )$

Sinussatz

${{\sin \alpha } \over a}={{\sin \beta } \over b}={{\sin \gamma } \over c}$

Cosinussatz

$a^{2}=b^{2}+c^{2}-2\cdot b\cdot c\cdot \cos \alpha$

$b^{2}=a^{2}+c^{2}-2\cdot a\cdot c\cdot \cos \beta$

$c^{2}=a^{2}+b^{2}-2\cdot a\cdot b\cdot \cos \gamma$

Grad und Radiant

• Grad: 360° entsprechen einem Vollwinkel
• Neugrad: 400g (gon) entsprechen einem Vollwinkel
• Bogenmaß/Radiant: 2$\pi$ entsprechen einem Vollwinkel

Umrechnung Grad in Bogenmaß

$b={{2\cdot \pi \cdot \alpha } \over 360^{\circ }}$

---

---

Von Trigonometrie

Winkelsumme

Bei einem Dreieck ist :α + β + γ = 180°.

Sinussatz

${\frac {b}{c}}={\frac {\sin \beta }{\sin \gamma }};\;\;\;\;{\frac {c}{a}}={\frac {\sin \gamma }{\sin \alpha }};\;\;\;\;{\frac {a}{b}}={\frac {\sin \alpha }{\sin \beta }}$

$a:\sin \alpha =b:\sin \beta =c:\sin \gamma$

$a:b:c=\sin \alpha :\sin \beta :\sin \gamma$ (Verhältnisgleichung)

Siehe auch: Sinussatz

Kosinussatz

$a^{2}=b^{2}+c^{2}-2bc\cos \alpha$

$b^{2}=c^{2}+a^{2}-2ca\cos \beta$

$c^{2}=a^{2}+b^{2}-2ab\cos \gamma$

$\cos \alpha ={\frac {b^{2}+c^{2}-a^{2}}{2bc}}$

$\cos \beta ={\frac {c^{2}+a^{2}-b^{2}}{2ca}}$

$\cos \gamma ={\frac {a^{2}+b^{2}-c^{2}}{2ab}}$

$a^{2}+bc\cos \alpha =b^{2}+ca\cos \beta =c^{2}+ab\cos \gamma ={\frac {a^{2}+b^{2}+c^{2}}{2}}$

Siehe auch: Cosinussatz

Projektionssatz

$a=b\cos \gamma +c\cos \beta$

$b=c\cos \alpha +a\cos \gamma$

$c=a\cos \beta +b\cos \alpha$

Die Mollweideschen Formeln

${\frac {b+c}{a}}={\frac {\cos {\frac {\beta -\gamma }{2}}}{\sin {\frac {\alpha }{2}}}};\;\;\;\;{\frac {b-c}{a}}={\frac {\sin {\frac {\beta -\gamma }{2}}}{\cos {\frac {\alpha }{2}}}}$

Analoge Formeln gelten für (c + a)/b, (c - a)/b, (a + b)/c und (a - b)/c.

Tangenssatz

${\frac {b+c}{b-c}}={\frac {\tan {\frac {\alpha +\beta }{2}}}{\tan {\frac {\alpha -\beta }{2}}}}={\frac {\cot {\frac {\gamma }{2}}}{\tan {\frac {\alpha -\beta }{2}}}}$

Analoge Formeln gelten für (c + a)/(c - a) und (a + b)/(a - b).

Siehe auch: Tangenssatz

Formeln mit dem halben Umfang

Im folgenden bedeutet s immer die Hälfte des Umfangs des Dreiecks ABC, also $s={\frac {a+b+c}{2}}$.

$s-a={\frac {b+c-a}{2}};\;\;\;\;s-b={\frac {c+a-b}{2}};\;\;\;\;s-c={\frac {a+b-c}{2}}$

$\left(s-b\right)+\left(s-c\right)=a$

$\left(s-c\right)+\left(s-a\right)=b$

$\left(s-a\right)+\left(s-b\right)=c$

$\left(s-a\right)+\left(s-b\right)+\left(s-c\right)=s$

$\sin {\frac {\alpha }{2}}={\sqrt {\frac {\left(s-b\right)\left(s-c\right)}{bc}}};\;\;\;\;\sin {\frac {\beta }{2}}={\sqrt {\frac {\left(s-c\right)\left(s-a\right)}{ca}}};\;\;\;\;\sin {\frac {\gamma }{2}}={\sqrt {\frac {\left(s-a\right)\left(s-b\right)}{ab}}}$

$\cos {\frac {\alpha }{2}}={\sqrt {\frac {s\left(s-a\right)}{bc}}};\;\;\;\;\cos {\frac {\beta }{2}}={\sqrt {\frac {s\left(s-b\right)}{ca}}};\;\;\;\;\cos {\frac {\gamma }{2}}={\sqrt {\frac {s\left(s-c\right)}{ab}}}$

$\tan {\frac {\alpha }{2}}={\sqrt {\frac {\left(s-b\right)\left(s-c\right)}{s\left(s-a\right)}}};\;\;\;\;\tan {\frac {\beta }{2}}={\sqrt {\frac {\left(s-c\right)\left(s-a\right)}{s\left(s-b\right)}}};\;\;\;\;\tan {\frac {\gamma }{2}}={\sqrt {\frac {\left(s-a\right)\left(s-b\right)}{s\left(s-c\right)}}}$

$s=4r\cos {\frac {\alpha }{2}}\cos {\frac {\beta }{2}}\cos {\frac {\gamma }{2}}$

$s-a=4r\cos {\frac {\alpha }{2}}\sin {\frac {\beta }{2}}\sin {\frac {\gamma }{2}}$

Analoge Formeln gelten für s-b und s-c.

Flächeninhalt und Umkreisradius

Der Flächeninhalt des Dreiecks ABC wird hier mit F bezeichnet (nicht, wie heute üblich, mit A, um eine Verwechselung mit der Dreiecksecke A auszuschließen):

Den Umkreisradius des Dreiecks ABC bezeichnen wir mit r.

[Es ist zu beachten, daß die hier benutzten Bezeichnungen r, ρ, ρ_a, ρ_b, ρ_c für den Umkreisradius, den Inkreisradius und die drei Ankreisradien von der vorwiegend im englischsprachigen Raum verbreiteten Bezeichnungsweise abweichen, bei der dieselben Größen R, r, r_a, r_b, r_c genannt werden.]

Heronsche Formel: $F={\sqrt {s\left(s-a\right)\left(s-b\right)\left(s-c\right)}}={\frac {1}{4}}{\sqrt {\left(a+b+c\right)\left(b+c-a\right)\left(c+a-b\right)\left(a+b-c\right)}}$

$F={\frac {1}{4}}{\sqrt {2\left(b^{2}c^{2}+c^{2}a^{2}+a^{2}b^{2}\right)-\left(a^{4}+b^{4}+c^{4}\right)}}$

$F={\frac {1}{2}}bc\sin \alpha ={\frac {1}{2}}ca\sin \beta ={\frac {1}{2}}ab\sin \gamma$

$F={\frac {1}{2}}ah_{a}={\frac {1}{2}}bh_{b}={\frac {1}{2}}ch_{c}$, wobei h_a, h_b und h_c die Längen der von A, B bzw. C ausgehenden Höhen des Dreiecks ABC sind.

$F=2r^{2}\sin \alpha \sin \beta \sin \gamma$

$F={\frac {abc}{4r}}$

$F=\rho s=\rho _{a}\left(s-a\right)=\rho _{b}\left(s-b\right)=\rho _{c}\left(s-c\right)$

$F={\sqrt {\rho \rho _{a}\rho _{b}\rho _{c}}}$

Erweiterter Sinussatz: ${\frac {a}{\sin \alpha }}={\frac {b}{\sin \beta }}={\frac {c}{\sin \gamma }}=2r$

$a=2r\sin \alpha$

$b=2r\sin \beta$

$c=2r\sin \gamma$

$r={\frac {abc}{4F}}$

In- und Ankreisradien

In diesem Abschnitt werden Formeln aufgelistet, in denen der Inkreisradius ρ und die Ankreisradien ρ_a, ρ_b und ρ_c des Dreiecks ABC vorkommen.

$\rho =\left(s-a\right)\tan {\frac {\alpha }{2}}=\left(s-b\right)\tan {\frac {\beta }{2}}=\left(s-c\right)\tan {\frac {\gamma }{2}}$

$\rho =4r\sin {\frac {\alpha }{2}}\sin {\frac {\beta }{2}}\sin {\frac {\gamma }{2}}=s\tan {\frac {\alpha }{2}}\tan {\frac {\beta }{2}}\tan {\frac {\gamma }{2}}$

$\rho =r\left(\cos \alpha +\cos \beta +\cos \gamma -1\right)$

$\rho ={\frac {F}{s}}={\frac {abc}{4rs}}$

$\rho ={\sqrt {\frac {\left(s-a\right)\left(s-b\right)\left(s-c\right)}{s}}}={\frac {1}{2}}{\sqrt {\frac {\left(b+c-a\right)\left(c+a-b\right)\left(a+b-c\right)}{a+b+c}}}$

$\rho ={\frac {a}{\cot {\frac {\beta }{2}}+\cot {\frac {\gamma }{2}}}}={\frac {b}{\cot {\frac {\gamma }{2}}+\cot {\frac {\alpha }{2}}}}={\frac {c}{\cot {\frac {\alpha }{2}}+\cot {\frac {\beta }{2}}}}$

Wichtige Ungleichung: $2\rho \leq r$; Gleichheit tritt nur dann ein, wenn Dreieck ABC gleichseitig ist.

$\rho _{a}=s\tan {\frac {\alpha }{2}}=\left(s-b\right)\tan {\frac {\gamma }{2}}=\left(s-c\right)\tan {\frac {\beta }{2}}$

$\rho _{a}=4r\sin {\frac {\alpha }{2}}\cos {\frac {\beta }{2}}\cos {\frac {\gamma }{2}}=\left(s-a\right)\tan {\frac {\alpha }{2}}\cot {\frac {\beta }{2}}\cot {\frac {\gamma }{2}}$

$\rho _{a}=r\left(-\cos \alpha +\cos \beta +\cos \gamma +1\right)$

$\rho _{a}={\frac {F}{s-a}}={\frac {abc}{4r\left(s-a\right)}}$

$\rho _{a}={\sqrt {\frac {s\left(s-b\right)\left(s-c\right)}{s-a}}}={\frac {1}{2}}{\sqrt {\frac {\left(a+b+c\right)\left(c+a-b\right)\left(a+b-c\right)}{b+c-a}}}$

Die Ankreise sind gleichberechtigt: Jede Formel für ρ_a gilt in analoger Form für ρ_b und ρ_c.

${\frac {1}{\rho }}={\frac {1}{\rho _{a}}}+{\frac {1}{\rho _{b}}}+{\frac {1}{\rho _{c}}}$

Höhen

Die Längen der von A, B bzw. C ausgehenden Höhen des Dreiecks ABC werden mit h_a, h_b und h_c bezeichnet.

$h_{a}=b\sin \gamma =c\sin \beta ={\frac {2F}{a}}=2r\sin \beta \sin \gamma$

$h_{b}=c\sin \alpha =a\sin \gamma ={\frac {2F}{b}}=2r\sin \gamma \sin \alpha$

$h_{c}=a\sin \beta =b\sin \alpha ={\frac {2F}{c}}=2r\sin \alpha \sin \beta$

$h_{a}={\frac {a}{\cot \beta +\cot \gamma }};\;\;\;\;\;h_{b}={\frac {b}{\cot \gamma +\cot \alpha }};\;\;\;\;\;h_{c}={\frac {c}{\cot \alpha +\cot \beta }}$

$F={\frac {1}{2}}ah_{a}={\frac {1}{2}}bh_{b}={\frac {1}{2}}ch_{c}$

${\frac {1}{h_{a}}}+{\frac {1}{h_{b}}}+{\frac {1}{h_{c}}}={\frac {1}{\rho }}={\frac {1}{\rho _{a}}}+{\frac {1}{\rho _{b}}}+{\frac {1}{\rho _{c}}}$

Hat das Dreieck ABC einen rechten Winkel bei C (ist also γ = 90°), dann gilt

$h_{c}={\frac {ab}{c}}$

$h_{a}=b$

$h_{b}=a$

Seitenhalbierende

Die Längen der von A, B bzw. C ausgehenden Seitenhalbierenden des Dreiecks ABC werden s_a, s_b und s_c genannt.

$s_{a}={\frac {1}{2}}{\sqrt {2b^{2}+2c^{2}-a^{2}}}={\frac {1}{2}}{\sqrt {b^{2}+c^{2}+2bc\cos \alpha }}={\sqrt {{\frac {a^{2}}{4}}+bc\cos \alpha }}$

$s_{b}={\frac {1}{2}}{\sqrt {2c^{2}+2a^{2}-b^{2}}}={\frac {1}{2}}{\sqrt {c^{2}+a^{2}+2ca\cos \beta }}={\sqrt {{\frac {b^{2}}{4}}+ca\cos \beta }}$

$s_{c}={\frac {1}{2}}{\sqrt {2a^{2}+2b^{2}-c^{2}}}={\frac {1}{2}}{\sqrt {a^{2}+b^{2}+2ab\cos \gamma }}={\sqrt {{\frac {c^{2}}{4}}+ab\cos \gamma }}$

$s_{a}^{2}+s_{b}^{2}+s_{c}^{2}={\frac {3}{4}}\left(a^{2}+b^{2}+c^{2}\right)$

Winkelhalbierende

Wir bezeichnen mit w_α, w_β und w_γ die Längen der von A, B bzw. C ausgehenden Winkelhalbierenden im Dreieck ABC.

$w_{\alpha }={\frac {2bc\cos {\frac {\alpha }{2}}}{b+c}}={\frac {2F}{a\cos {\frac {\beta -\gamma }{2}}}}$

$w_{\beta }={\frac {2ca\cos {\frac {\beta }{2}}}{c+a}}={\frac {2F}{b\cos {\frac {\gamma -\alpha }{2}}}}$

$w_{\gamma }={\frac {2ab\cos {\frac {\gamma }{2}}}{a+b}}={\frac {2F}{c\cos {\frac {\alpha -\beta }{2}}}}$

Literatur

• Lehrbuch und Übungsbuch Mathematik: Bd. 2 Planimetrie, Stereometrie und Trigonometrie der Ebene. Von Wolfgang Pauli (1991), ISBN 3-446-00755-5 , KNO-NR: 04 41 57 51

---

---

Von Trigonometrische Funktion

Definition

Ursprünglich als Seitenverhältnisse in rechtwinkligen Dreiecken und daher nur für Winkel von 0 bis 90 Grad definiert (siehe Sinus, Kosinus, Tangens), können die Winkelfunktionen als Sekanten- und Tangentenabschnitte am Einheitskreis auch auf größere Winkel erweitert werden. Vom Schnittpunkt des einen Winkelschenkels mit dem Einheitskreis werden die Lote auf die beiden Koordinatenachsen gefällt und liefern Sinus und Kosinus des Winkels. Die Tangenten in den Punkten x = 1 bzw. y = 1 schneiden den Schenkel ebenfalls und liefern dann in der Projektion auf die Achsen den Tangens und den Kotangens. Dabei muss der Schenkel gegebenenfalls rückwärts verlängert werden, um einen Schnittpunkt zu erzielen. Auf diese Weise können jedem Winkel von 0 bis 360 Grad Werte der Winkelfunktionen zugeordnet werden, die nun freilich auch negativ werden können (siehe Abbildung).

Die Vorzeichen der Winkelfunktionen in Abhängigkeit vom Quadranten gibt die folgende Tabelle an:

Quadrant	sin	cos	tan	cot
I	+	+	+	+
II	+	−	−	−
III	−	−	+	+
IV	−	+	−	−

Eine Tabelle spezieller Werte findet sich unter Reduktionsformeln.

Anwendung der trigonometrischen Funktionen

Hauptsächlich werden die trigonometrischen Funktionen im Vermessungswesen genutzt. Für eine Liste von Formeln zur Berechnung von Größen am Dreieck siehe den Artikel Dreiecksgeometrie.

Weiterhin sind sie in der Analysis und bei vielen Anwendungen der Physik wichtig. Es besteht eine enge Beziehung zur Exponentialfunktion, die besonders bei Funktionen komplexer Zahlen und in der Taylorreihe der Funktionen sichtbar wird.

Umkehrung der trigonometrischen Funktionen

In manchen Situationen werden die trigonometrischen Winkelfunktionen benötigt, um aus Seitenverhältnissen Winkel zu berechnen. Dazu werden die Arcus-Funktionen oder inverse Winkelfunktionen arcsin, arccos, arctan und arccot- die Umkehrfunktionen zu den trigonometrischen Funktionen - verwendet. Auf Taschenrechnern sind sie häufig (irreführenderweise) mit sin^-1 usw. bezeichnet, was die Umkehrung zu sin andeuten solle.

Die Arcus-Funktionen werden verwendet, um zu einem Seitenverhältnis den Winkel zu berechnen. Wegen der Symmetrie der trigonometrischen Funktionen ist bei ihnen zu klären, in dem Quadrant der gesuchte Winkel liegt.

• Siehe http://www.mathe-online.at/mathint/lexikon/i.html#inverseWinkelfunktionen

Funktionale Zusammenhänge

Gegenseitige Darstellung

Die trigonometrischen Funktionen lassen sich ineinander umwandeln oder gegenseitig darstellen. Es gelten folgende Zusammenhänge:

$\tan x={\frac {\sin x}{\cos x}}$

$\sin ^{2}x+\cos ^{2}x=1$

$1+\tan ^{2}x={\frac {1}{\cos ^{2}x}}=\sec ^{2}x$

$1+\cot ^{2}x={\frac {1}{\sin ^{2}x}}=\csc ^{2}x$

Mittels dieser Gleichungen lassen sich die drei vorkommenden Funktionen durch eine der beiden anderen darstellen:

$\sin x={\sqrt {1-\cos ^{2}x}}\quad f{\ddot {u}}r\quad x\in \left[0^{\circ };\;180^{\circ }\right]$

$\sin x=-{\sqrt {1-\cos ^{2}x}}\quad f{\ddot {u}}r\quad x\in \left[180^{\circ };\;360^{\circ }\right]$

$\sin x={\frac {\tan x}{\sqrt {1+\tan ^{2}x}}}\quad f{\ddot {u}}r\quad x\in \left[0^{\circ };\;90^{\circ }\right]\cup \left[270^{\circ };\;360^{\circ }\right]$

$\sin x=-{\frac {\tan x}{\sqrt {1+\tan ^{2}x}}}\quad f{\ddot {u}}r\quad x\in \left[90^{\circ };\;270^{\circ }\right]$

$\cos x={\sqrt {1-\sin ^{2}x}}\quad f{\ddot {u}}r\quad x\in \left[0^{\circ };\;90^{\circ }\right]\cup \left[270^{\circ };\;360^{\circ }\right]$

$\cos x=-{\sqrt {1-\sin ^{2}x}}\quad f{\ddot {u}}r\quad x\in \left[90^{\circ };\;270^{\circ }\right]$

$\cos x={\frac {1}{\sqrt {1+\tan ^{2}x}}}\quad f{\ddot {u}}r\quad x\in \left[0^{\circ };\;90^{\circ }\right]\cup \left[270^{\circ };\;360^{\circ }\right]$

$\cos x=-{\frac {1}{\sqrt {1+\tan ^{2}x}}}\quad f{\ddot {u}}r\quad x\in \left[90^{\circ };\;270^{\circ }\right]$

$\tan x={\frac {\sqrt {1-\cos ^{2}x}}{\cos x}}\quad f{\ddot {u}}r\quad x\in \left[0^{\circ };\;180^{\circ }\right]$

$\tan x=-{\frac {\sqrt {1-\cos ^{2}x}}{\cos x}}\quad f{\ddot {u}}r\quad x\in \left[180^{\circ };\;360^{\circ }\right]$

$\tan x={\frac {\sin x}{\sqrt {1-\sin ^{2}x}}}\quad f{\ddot {u}}r\quad x\in \left[0^{\circ };\;90^{\circ }\right]\cup \left[270^{\circ };\;360^{\circ }\right]$

$\tan x=-{\frac {\sin x}{\sqrt {1-\sin ^{2}x}}}\quad f{\ddot {u}}r\quad x\in \left[90^{\circ };\;270^{\circ }\right]$

Vorzeichen der Winkelfunktionen

$\sin x>0\quad f{\ddot {u}}r\quad x\in \left]0^{\circ };\;180^{\circ }\right[$

$\sin x<0\quad f{\ddot {u}}r\quad x\in \left]180^{\circ };\;360^{\circ }\right[$

$\cos x>0\quad f{\ddot {u}}r\quad x\in \left]0^{\circ };\;90^{\circ }\right[\cup \left]270^{\circ };\;360^{\circ }\right[$

$\cos x<0\quad f{\ddot {u}}r\quad x\in \left]90^{\circ };\;270^{\circ }\right[$

$\tan x>0\quad f{\ddot {u}}r\quad x\in \left]0^{\circ };\;90^{\circ }\right]\cup \left[180^{\circ };\;270^{\circ }\right[$

$\tan x<0\quad f{\ddot {u}}r\quad x\in \left]90^{\circ };\;180^{\circ }\right]\cup \left[270^{\circ };\;360^{\circ }\right[$

Die Vorzeichen von cot, sec und csc stimmen überein mit denen ihrer Kehrwertfunktionen tan, cos bzw. sin.

Symmetrien

Die trigonometrischen Funktionen haben einfache Symmetrien:

$\sin(-x)=-\sin x$

$\cos(-x)=\cos x$

$\tan(-x)=-\tan x$

$\cot(-x)=-\cot x$

$\sec(-x)=\sec x$

$\csc(-x)=-\csc x$

Additionstheoreme

Weiterhin sind die Additionstheoreme nützlich:

$\sin(x+y)=\sin x\;\cos y+\sin y\;\cos x$

$\sin(x-y)=\sin x\;\cos y-\sin y\;\cos x$

$\cos(x+y)=\cos y\;\cos x-\sin x\;\sin y$

$\cos(x-y)=\cos y\;\cos x+\sin x\;\sin y$

$\tan(x+y)={\frac {\tan x+\tan y}{1-\tan x\;\tan y}}={\frac {\sin(x+y)}{\cos(x+y)}}$

$\tan(x-y)={\frac {\tan x-\tan y}{1+\tan x\;\tan y}}={\frac {\sin(x-y)}{\cos(x-y)}}$

$\cot \left(x+y\right)={\frac {\cot x\cot y-1}{\cot x+\cot y}}={\frac {\cos(x+y)}{\sin(x+y)}}$

$\cot \left(x-y\right)={\frac {-\left(\cot x\cot y+1\right)}{\cot x-\cot y}}={\frac {\cos(x-y)}{\sin(x-y)}}$

$\sin(x+y)\sin(x-y)=\cos ^{2}y-\cos ^{2}x$

$\cos(x+y)\cos(x-y)=\cos ^{2}y-\sin ^{2}x$

Für x = y folgen hieraus die

Doppelwinkelfunktionen

$\sin(2\;x)=2\sin x\;\cos x={\frac {2\tan x}{1+\tan ^{2}x}}$

$\cos(2\;x)=\cos ^{2}x-\sin ^{2}x=1-2\sin ^{2}x=2\cos ^{2}x-1={\frac {1-\tan ^{2}x}{1+\tan ^{2}x}}$

$\tan(2\;x)={\frac {2\tan x}{1-\tan ^{2}x}}={\frac {2}{\cot x-\tan x}}$

$\cot(2\;x)={\frac {\cot ^{2}x-1}{2\cot x}}={\frac {\cot x-\tan x}{2}}$

Winkelfunktionen für weitere Vielfache

$\sin(3\;x)=3\sin x-4\sin ^{3}x$

$\sin(4\;x)=8\sin x\;\cos ^{3}x-4\sin x\;\cos x$

$\sin(5\;x)=16\sin x\;\cos ^{4}x-12\sin x\;\cos ^{2}x+\sin x$

$\sin(n\;x)=n\;\sin x\;\cos ^{n-1}x-{n \choose 3}\sin ^{3}x\;\cos ^{n-3}x+{n \choose 5}\sin ^{5}x\;\cos ^{n-5}x\;-\;+\;\dots$

$\cos(3\;x)=4\cos ^{3}x-3\cos x$

$\cos(4\;x)=8\cos ^{4}x-8\cos ^{2}x+1$

$\cos(5\;x)=16\cos ^{5}x-20\cos ^{3}x+5\cos x$

$\cos(n\;x)=\cos ^{n}x-{n \choose 2}\sin ^{2}x\;\cos ^{n-2}x+{n \choose 4}\sin ^{4}x\;\cos ^{n-4}x\;-\;+\;\dots$

$\tan(3\;x)={\frac {3\tan x-\tan ^{3}x}{1-3\tan ^{2}x}}$

$\tan(4\;x)={\frac {4\tan x-4\tan ^{3}x}{1-6\tan ^{2}x+\tan ^{4}x}}$

$\cot(3\;x)={\frac {\cot ^{3}x-3\cot x}{3\cot ^{2}x-1}}$

$\cot(4\;x)={\frac {\cot ^{4}x-6\cot ^{2}x+1}{4\cot ^{3}x-4\cot x}}$

Halbwinkelformeln

Zur Berechnung des Funktionswertes des halben Arguments dienen die Halbwinkelformeln:

$\sin({\frac {x}{2}})=\pm {\sqrt {\frac {1-\cos(x)}{2}}}\quad f{\ddot {u}}r\quad x\in \left[0^{\circ };\;360^{\circ }\right]$

$\cos({\frac {x}{2}})=\pm {\sqrt {\frac {1+\cos(x)}{2}}}\quad f{\ddot {u}}r\quad x\in \left[-180^{\circ };\;180^{\circ }\right]$

$\tan({\frac {x}{2}})=\pm {\sqrt {\frac {1-\cos(x)}{1+\cos(x)}}}={\frac {1-\cos(x)}{\sin(x)}}={\frac {\sin(x)}{1+\cos(x)}}\quad f{\ddot {u}}r\quad x\in \left(-180^{\circ };\;180^{\circ }\right)$

$\cot({\frac {x}{2}})=\pm {\sqrt {\frac {1+\cos(x)}{1-\cos(x)}}}={\frac {1+\cos(x)}{\sin(x)}}={\frac {\sin(x)}{1-\cos(x)}}\quad f{\ddot {u}}r\quad x\in \left(-180^{\circ };\;180^{\circ }\right)$

Identitäten

Aus den Additionstheoremen lassen sich Identitäten ableiten, mit denen die Summe zweier trigonometrischer Funktionen als Produkt aufgefasst werden kann:

$\sin x+\sin y=2\sin {\frac {x+y}{2}}\cos {\frac {x-y}{2}}$

$\sin x-\sin y=2\cos {\frac {x+y}{2}}\sin {\frac {x-y}{2}}$

$\cos x+\cos y=2\cos {\frac {x+y}{2}}\cos {\frac {x-y}{2}}$

$\cos x-\cos y=-2\sin {\frac {x+y}{2}}\sin {\frac {x-y}{2}}$

$\tan x+\tan y={\frac {\sin(x+y)}{\cos x\cos y}}$

$\tan x-\tan y={\frac {\sin(x-y)}{\cos x\cos y}}$

$\cot x+\cot y={\frac {\sin(x+y)}{\sin x\sin y}}$

$\cot x-\cot y={\frac {-\sin(x-y)}{\sin x\sin y}}$

Produkte der Winkelfunktionen

$\sin x\;\sin y={\frac {1}{2}}\left[\cos(x-y)-\cos(x+y)\right]$

$\cos x\;\cos y={\frac {1}{2}}\left[\cos(x-y)+\cos(x+y)\right]$

$\sin x\;\cos y={\frac {1}{2}}\left[\sin(x-y)+\sin(x+y)\right]$

$\tan x\;\tan y={\frac {\tan x+\tan y}{\cot x+\cot y}}=-{\frac {\tan x-\tan y}{\cot x-\cot y}}$

$\cot x\;\cot y={\frac {\cot x+\cot y}{\tan x+\tan y}}=-{\frac {\cot x-\cot y}{\tan x-\tan y}}$

$\tan x\;\cot y={\frac {\tan x+\cot y}{\cot x+\tan y}}=-{\frac {\tan x-\cot y}{\cot x-\tan y}}$

$\sin x\;\sin y\;\sin z={\frac {1}{4}}\left[\sin(x+y-z)+\sin(y+z-x)+\sin(z+x-y)-\sin(x+y+z)\right]$

$\cos x\;\cos y\;\cos z={\frac {1}{4}}\left[\cos(x+y-z)+\cos(y+z-x)+\cos(z+x-y)+\cos(x+y+z)\right]$

$\sin x\;\sin y\;\cos z={\frac {1}{4}}\left[-\cos(x+y-z)+\cos(y+z-x)+\cos(z+x-y)-\cos(x+y+z)\right]$

$\sin x\;\cos y\;\cos z={\frac {1}{4}}\left[\sin(x+y-z)-\sin(y+z-x)+\sin(z+x-y)+\sin(x+y+z)\right]$

Potenzen der Winkelfunktionen

$\sin ^{2}x={\frac {1}{2}}\ \left[1-\cos(2\ x)\right]$

$\cos ^{2}x={\frac {1}{2}}\ \left[1+\cos(2\ x)\right]$

$\tan ^{2}x={\frac {1-\cos(2\ x)}{1+\cos(2\ x)}}$

$\sin ^{3}x={\frac {1}{4}}\ \left[3\sin x-\sin(3\ x)\right]$

$\cos ^{3}x={\frac {1}{4}}\ \left[3\cos x+\cos(3\ x)\right]$

$\sin ^{4}x={\frac {1}{8}}\ \left[\cos(4\ x-4\cos(2\ x)+3\right]$

$\cos ^{4}x={\frac {1}{8}}\ \left[\cos(4\ x+4\cos(2\ x)+3\right]$

$\sin ^{5}x={\frac {1}{16}}\ \left[10\sin x-5\sin(3\ x)+\sin(5\ x)\right]$

$\cos ^{5}x={\frac {1}{16}}\ \left[10\cos x+5\cos(3\ x)+\cos(5\ x)\right]$

$\sin ^{6}x={\frac {1}{32}}\ \left[10-15\cos(2\ x)+6\cos(4\ x)-\cos(6\ x)\right]$

$\cos ^{6}x={\frac {1}{32}}\ \left[10+15\cos(2\ x)+6\cos(4\ x)+\cos(6\ x)\right]$

Reduktionsformeln

Es gibt Reduktionsformeln, mit denen man das Argument einer Winkelfunktion in das Intervall [0, 90°] bzw. [0, π/4] bringen kann.

Siehe auch: Zusammenhang mit den Hyperbelfunktionen, Quadrant

Weitere Formeln

Die folgenden Formeln folgen nach mehr oder weniger langen Termumformungen aus α + β + γ = 180°, gelten also allgemein für drei beliebige Winkel α, β und γ mit α + β + γ = 180°, solange die die in den Formeln vorkommenden Funktionen wohldefiniert sind (letzteres betrifft nur die Formeln, in denen Tangense und Kotangense vorkommen).

$\tan \alpha +\tan \beta +\tan \gamma =\tan \alpha \tan \beta \tan \gamma$

$\cot \beta \cot \gamma +\cot \gamma \cot \alpha +\cot \alpha \cot \beta =1$

$\cot {\frac {\alpha }{2}}+\cot {\frac {\beta }{2}}+\cot {\frac {\gamma }{2}}=\cot {\frac {\alpha }{2}}\cot {\frac {\beta }{2}}\cot {\frac {\gamma }{2}}$

$\tan {\frac {\beta }{2}}\tan {\frac {\gamma }{2}}+\tan {\frac {\gamma }{2}}\tan {\frac {\alpha }{2}}+\tan {\frac {\alpha }{2}}\tan {\frac {\beta }{2}}=1$

$\sin \alpha +\sin \beta +\sin \gamma =4\cos {\frac {\alpha }{2}}\cos {\frac {\beta }{2}}\cos {\frac {\gamma }{2}}$

$-\sin \alpha +\sin \beta +\sin \gamma =4\cos {\frac {\alpha }{2}}\sin {\frac {\beta }{2}}\sin {\frac {\gamma }{2}}$

$\cos \alpha +\cos \beta +\cos \gamma =4\sin {\frac {\alpha }{2}}\sin {\frac {\beta }{2}}\sin {\frac {\gamma }{2}}+1$

$-\cos \alpha +\cos \beta +\cos \gamma =4\sin {\frac {\alpha }{2}}\cos {\frac {\beta }{2}}\cos {\frac {\gamma }{2}}-1$

$\sin \left(2\alpha \right)+\sin \left(2\beta \right)+\sin \left(2\gamma \right)=4\sin \alpha \sin \beta \sin \gamma$

$-\sin \left(2\alpha \right)+\sin \left(2\beta \right)+\sin \left(2\gamma \right)=4\sin \alpha \cos \beta \cos \gamma$

$\cos \left(2\alpha \right)+\cos \left(2\beta \right)+\cos \left(2\gamma \right)=-4\cos \alpha \cos \beta \cos \gamma -1$

$-\cos \left(2\alpha \right)+\cos \left(2\beta \right)+\cos \left(2\gamma \right)=-4\cos \alpha \sin \beta \sin \gamma +1$

$\sin ^{2}\alpha +\sin ^{2}\beta +\sin ^{2}\gamma =2\cos \alpha \cos \beta \cos \gamma +2$

$-\sin ^{2}\alpha +\sin ^{2}\beta +\sin ^{2}\gamma =2\cos \alpha \sin \beta \sin \gamma$

$\cos ^{2}\alpha +\cos ^{2}\beta +\cos ^{2}\gamma =-2\cos \alpha \cos \beta \cos \gamma +1$

$-\cos ^{2}\alpha +\cos ^{2}\beta +\cos ^{2}\gamma =-2\cos \alpha \sin \beta \sin \gamma +1$

$-\sin ^{2}\left(2\alpha \right)+\sin ^{2}\left(2\beta \right)+\sin ^{2}\left(2\gamma \right)=-2\cos \left(2\alpha \right)\sin \left(2\beta \right)\sin \left(2\gamma \right)$

$-\cos ^{2}\left(2\alpha \right)+\cos ^{2}\left(2\beta \right)+\cos ^{2}\left(2\gamma \right)=2\cos \left(2\alpha \right)\sin \left(2\beta \right)\sin \left(2\gamma \right)+1$

Reihenentwicklung

Der Sinus (blau) verglichen mit seiner Taylorreihe bis x⁷ (pink)

Bitte beachten: Hier, wie auch sonst in der Analysis, ist es wichtig, dass alle Winkel im Bogenmaß angegeben werden.

Man kann zeigen, dass der Cosinus die Ableitung des Sinus darstellt und die Ableitung des Cosinus der negative Sinus ist. Hat man diese Ableitungen, kann man die Taylorreihe entwickeln und zeigen, dass die folgenden Identitäten für alle x aus den reellen Zahlen gelten:

$\sin(x)=x-{\frac {x^{3}}{3!}}+{\frac {x^{5}}{5!}}-{\frac {x^{7}}{7!}}+\;-\;\dots \;=\;\sum _{n=0}^{\infty }{\frac {(-1)^{n}}{(2n+1)!}}x^{2n+1}\quad f{\ddot {u}}r\quad |x|<\infty$

$\cos(x)=1-{\frac {x^{2}}{2!}}+{\frac {x^{4}}{4!}}-{\frac {x^{6}}{6!}}+\;-\;\dots \;=\;\sum _{n=0}^{\infty }{\frac {(-1)^{n}}{(2n)!}}x^{2n}\qquad \quad f{\ddot {u}}r\quad |x|<\infty$

$\tan(x)=x+{\frac {1}{3}}x^{3}+{\frac {2}{15}}x^{5}+{\frac {17}{315}}x^{7}+{\frac {62}{2835}}x^{9}+\;\dots \;=\;\sum _{n=0}^{\infty }{\frac {2^{2n}(2^{2n}-1)B_{n}}{(2n)!}}\quad f{\ddot {u}}r\quad |x|<{\frac {\pi }{2}}$

$\cot(x)={\frac {1}{x}}-{\frac {1}{3}}x-{\frac {1}{45}}x^{3}-{\frac {2}{945}}x^{5}-{\frac {1}{4725}}x^{7}-\;\dots \qquad f{\ddot {u}}r\quad 0<|x|<\pi$

Von Dreieck

In der Geometrie werden die Eckpunkte des Dreiecks in der Regel mit A, B und C bezeichnet. Die Seite, die einer Ecke gegenüberliegt, wird analog a, b bzw. c genannt. Damit liegt z.B. die Seite a dem Eckpunkt A gegenüber, verbindet also die Punkte B und C. Die Winkel werden α, β und γ genannt; α ist der Winkel am Eckpunkt A, usw.

• Die Summe der Winkel in einem planaren (ebenen) Dreieck beträgt immer 180°.
• Die Summe zweier Seiten eines Dreieck ist immer größer als die dritte Seite.

Diese intuitiv einsichtigen Eigenschaften ebener Dreiecke folgen aus den Axiomen der Euklidischen Geometrie.

Berechnung eines beliebigen Dreiecks

Hat man von einem beliebigen Dreieck drei Angaben (Seiten bzw. Winkel), kann man die 3 fehlenden Angaben berechnen. Die 5 Auflösungsfälle werden symbolisch bezeichnet: SSS, SSW, SWS, SWW, WSW.
Der 6.Fall WWW ist bei ebenen Dreiecken nicht lösbar, weil es de facto nur 2 Angaben sind (α+β+γ = 180°). Ohne gegebene Seite ist zwar die Form des Dreiecks gegeben, seine Größe bleibt aber offen.

Für Berechnungen sind der Sinussatz und der Kosinussatz am wichtigsten; zusätzlich kennt man den Projektionssatz sowie Tangenten- und Halbwinkelsätze.
Den Sinussatz gibt es in 3 Varianten, von denen sich aber die dritte aus den beiden anderen ergibt:

${\frac {a}{sin\alpha }}={\frac {b}{sin\beta }}={\frac {c}{sin\gamma }}$

Der Kosinussatz ist eine verallgemeinerte Form des "Pythagoras", mit dem sich die Seiten eines beliebigen Dreiecks berechnen lassen:

$a^{2}=b^{2}+c^{2}-2bc*\cos \alpha$

$b^{2}=a^{2}+c^{2}-2ac*\cos \beta$

$c^{2}=a^{2}+b^{2}-2ab*\cos \gamma$

Da für einen Winkel γ = 90° (bzw. ${\frac {\pi }{2}}$) der Cosinus γ = 0 ist, gilt für ein rechtwinkliges Dreieck die Formel

$c^{2}=a^{2}+b^{2}$

allgemeines Dreieck

Umfang:	$u=8r*\cos {\frac {\alpha }{2}}*\cos {\frac {\beta }{2}}*\cos {\frac {\gamma }{2}}$
Inkreisradius:	$\rho =4r*\sin {\frac {\alpha }{2}}*\sin {\frac {\beta }{2}}*\sin {\frac {\gamma }{2}}$
Umkreisradius:	$r={\frac {a}{2*\sin \ \alpha }}={\frac {b}{2*\sin \ \beta }}={\frac {c}{2*\sin \ \gamma }}$
Höhenformeln:	$h_{a}=c*\sin \ \beta =b*\sin \ \gamma$
	$h_{b}=a*\sin \ \gamma =c*\sin \ \alpha$
	$h_{c}=b*\sin \ \alpha =a*\sin \ \beta$
Flächeninhalt:	$A={\frac {1}{2}}ah_{a}={\frac {1}{2}}bh_{b}={\frac {1}{2}}ch_{c}$

Dreiecksarten

Übersicht der unterschiedlichen Arten von Dreiecken

Dreiecksarten	unregelmäßig Kein Winkel und keine Seite sind gleichgroß.	gleichschenklig Zwei Seiten und zwei Winkel sind gleichgroß	gleichseitig Alle Winkel und Seiten sind gleichgroß.
spitzwinklig Alle Winkel sind spitze Winkel, d.h. alle Winkel sind <90°.			Gleichseitiges Dreieck
rechtwinklig Ein Winkel ist ein rechter Winkel.			nicht in der Ebene möglich, da dort die winkelsumme 180° sein muss. Aber als Dreieck auf einer Kugelfläche möglich.
stumpfwinklig Ein Winkel ist ein stumpfer Winkel (>90°).			in der Ebene unmöglich

Das gleichseitige Dreieck

Gleichseitiges Dreieck

Eigenschaften

• Bei einem gleichseitigen Dreieck sind alle drei Seiten gleich lang und alle drei Innenwinkel gleich groß. Aus diesem Grund gehört das gleichseitige Dreieck auch zu den regelmäßigen Polygonen.
• Jeder Winkel eines gleichseitigen Dreiecks beträgt 60°.
• Das gleichseitige Dreieck zählt zu den spitzwinkligen Dreiecken, weil alle drei Winkel kleiner als 90° sind.
• Außerdem ist das gleichseitige Dreieck auch ein gleichschenkliges Dreieck.
• Alle gleichseitigen Dreiecke sind zueinander ähnlich.
• Mittelsenkrechte, Winkelhalbierende, Seitenhalbierende und Höhe zu einer Seite fallen bei einem gleichseitigen Dreieck jeweils zusammen. Entsprechendes gilt für den Umkreismittelpunkt, den Inkreismittelpunkt, den Schwerpunkt und den Höhenschnittpunkt des gleichseitigen Dreiecks.

Formeln

Die Seitenlänge des gleichseitigen Dreiecks wird mit a bezeichnet.

Fläche A	$A={\frac {\sqrt {3}}{4}}a^{2}$
Höhe h	$h={\frac {\sqrt {3}}{2}}a$
Umkreisradius r	$r={\frac {\sqrt {3}}{3}}a={\frac {1}{\sqrt {3}}}a$
Inkreisradius ρ	$\rho ={\frac {\sqrt {3}}{6}}a$
Umfang u	$u=3*a$

Das gleichschenklige Dreieck

Links ein gleichschenkliges, rechts ein gleichseitiges Dreieck

Eigenschaften

• Bei einem gleichschenkligen Dreieck sind wenigstens zwei Seiten gleich lang und die jeweils gegenüber liegenden Winkel gleich groß.
• Die beiden gleich langen Seiten bezeichnet man als Schenkel, die dritte als Basis.
• Die gleich großen Winkel, die den Schenkeln gegenüber liegen, heißen Basiswinkel.
• Der Punkt, an dem beide Schenkel zusammentreffen, nennt man Spitze.
• In einem gleichschenkligen Dreieck sind die Mittelsenkrechte zur Basis, die Winkelhalbierende des Winkels an der Spitze, die Seitenhalbierende der Basis und die Höhe zur Basis identisch.
• Das gleichseitige Dreieck lässt sich als eine spezielle Form des gleichschenkligen Dreiecks sehen, bei der jede Seite gleichzeitig Schenkel und Basis ist und jede Ecke des Dreiecks als Spitze bezeichnet werden kann.

Das rechtwinklige Dreieck

Eigenschaften

• Ein rechtwinkliges Dreieck besitzt einen 90°-Winkel, auch rechter Winkel genannt.
• Die längste Seite des Dreiecks liegt dem rechten Winkel gegenüber und wird Hypotenuse genannt.
• Die beiden anderen Seiten heißen Katheten.

Satz des Pythagoras	$c^{2}=a^{2}+b^{2}$
Kathetensatz von Euklid	$a^{2}=c*p$
	$b^{2}=c*q$
Höhensatz von Euklid	$h^{2}=p*q$

Bei Kenntnis zwei der Angaben (a, b, c, p, q und h) lassen sich die fehlenden 3 anderen Werte aus den, in der Tabelle aufgeführten Formeln berechnen.

Die Längen der drei Seiten werden durch den Satz des Pythagoras in Beziehung gebracht: Das Quadrat der Länge der Hypotenuse (in der Grafik als c bezeichnet) gleicht der Summe der Quadrate der Längen der Katheten (a und b).

In Bezug auf die spitzen Winkel des Dreiecks spricht man von der Ankathete des Winkels als die dem Winkel anliegende Kathete und von der Gegenkathete als die dem Winkel gegenüberliegende Kathete. Durch das Verhältnis zwischen Katheten und Hypotenuse lässt sich auch ein Winkel im rechtwinkligen Dreieck eindeutig bestimmen.

Rechtwinkliges Dreieck mit den rechten Winkel im Punkt C

Funktion	Berechnung
Der Sinus des Winkels α ist dabei als das Verhältnis zwischen der Gegenkathete (hier: a) und der Hypotenuse (hier: c) definiert.	$\sin \alpha ={\frac {a}{c}}$
Der Kosinus des Winkels α ist das Verhältnis zwischen der Ankathete (hier: b) und der Hypotenuse.	$\cos \alpha ={\frac {b}{c}}$
Der Tangens ist durch das Verhältnis zwischen Gegenkathete und Ankathete gegeben.	$\tan \alpha ={\frac {a}{b}}$
Der Kotangens ist das Verhältnis zwischen Ankathete und Gegenkathete, und ist damit der Kehrwert des Tangens.	$\cot \alpha ={\frac {b}{a}}={\frac {1}{\tan \alpha }}$
Der Sekans ist das Verhältnis der Hypotenuse zur Ankathete, also der Kehrwert des Kosinus.	$\sec \alpha ={\frac {c}{b}}={\frac {1}{\cos \alpha }}$
Der Kosekans ist das Verhältnis der Hypotenuse zur Gegenkathete, d. h. der Kehrwert des Sinus.	$\csc \alpha ={\frac {c}{a}}={\frac {1}{\sin \alpha }}$

Diese sechs Funktionen werden Winkelfunktionen oder trigonometrische Funktionen genannt; im schulischen Kanon werden diese jedoch meistens auf die ersten drei reduziert (diese sind auch die geläufigsten, die anderen sind seltener von Bedeutung).

Dreiecke der Nichteuklidschen Geometrie

Sphärische Dreiecke

Dreiecke auf der Kugel nennt man sphärisch), wobei die 3 Seiten Teile von Großkreisen sind. Ihre Seitenlänge wird nicht in der Dimension einer Länge angegeben (Meter, cm usw.), sondern als zugehöriger Winkel im Kugelmittelpunkt.

Sphärisches Dreieck (Kugeldreieck)

Ein sphärisches Dreieck hat eine Winkelsumme größer als 180°, wobei der "Überschuss" sphärischer Exzess heißt und in Formeln meist als ε bezeichnet wird: $\alpha +\beta +\gamma =180^{\circ }+\epsilon$.

Der Exzess hängt direkt mit dem Flächeninhalt des Dreiecks zusammen (ε = F / R² , bzw. in Grad ε = 180°.F / R²π), worin R den Kugelradius und π die Kreiszahl 3,14159... bedeutet.
Der maximale Exzess von 360° tritt beim größtmöglichen "Dreieck" auf, das die halbe Kugeloberfläche umspannt: mit 3 gestreckten Winkeln hat es eine Winkelsumme von 3mal 180° und ε = 540° - 180°.

Sphärische Dreiecke können analog den ebenen Dreiecken berechnet werden, wofür es z.B. den spärischen Sinussatz, den Cosinussatz, den Projektionssatz und verschiedene Halbwinkelsätze gibt - siehe Sphärische Trigonometrie.

Sphärisches Zweieck

Sphärisches Zweieck

Sphärisches Zweieck: für manche Berechnungen auf der Sphäre - z.B. auf der Himmelskugel - sind auch Zweiecke nützlich. Die Formeln ergeben sich als Sonderfall des Dreiecks.

Hyperbolische Dreiecke

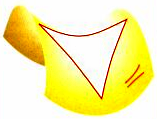
Sattelfläche und geodätisches Dreieck

Zur nichteuklidischen Geometrie - in der das Parallelen-Axiom nicht gilt - zählen z.B. auch Dreiecke auf einer Sattelfläche. Während eine Kugel überall konvex gekrümmt ist, haben Sattel- und andere hyperbolische Flächen sowohl konvexe als auch konkave Krümmung (ihr Produkt, das Krümmungsmaß, ist negativ).

Entsprechend ist auch der Exzess negativ - d.h. die Winkelsumme eines Dreiecks auf einer Sattelfläche ist kleiner als 180°.

 ---

Die Kongruenzsätze machen Aussagen über die Dreiecksgrößen (Seitenlänge, Winkel), die notwendig sind, um ein Dreieck eindeutig zu bestimmen.
In der Trigonometrie, einem Teilgebiet der Mathematik, spielen Dreiecke die wesentliche Rolle. Siehe dazu insbesondere Dreiecksgeometrie.

Oft auftretende Dreiecksgrößen

• die Fläche
• die Höhen
• die Seitensymmetralen
• die Winkelsymmetralen
• die Seitenhalbierenden
• der Inkreis
• der Umkreis

Interessant sind auch die Schnittpunkte dieser Linien bzw. die Mittelpunkte der Kreise, die als ausgezeichnete Punkte des Dreiecks bekannt sind.

Sätze rund um das Dreieck

• Kongruenzsätze
• Thaleskreis
• Ausgezeichnete Punkte im Dreieck
• Südpolsatz
• Sinussatz
• Satz von Pythagoras, Kosinussatz
• Satz von Menelaos
• Satz von Ceva
• Satz von Stewart
• Satz des Heron (Fläche aus drei Seiten berechnen)
• Eulersche Gerade
• Feuerbachkreis
• Simsonsche Gerade
• Symmedianen und Lemoinepunkt
• Trigonometrie

Weblinks

• Bilder verschiedener Dreiecksarten
• Lösungsprogramm in JavaScript und Lösungsalgorithmus für Dreiecke auf www.ArsTechnica.de